A.A.Kubasov (kubasov@comp.chem.msu.su),
V.S.Lyutsarev (lvs@comp.chem.msu.su)
and
K.V.Ermakov (ekv@comp.chem.msu.su),
Chemical Faculty,
Moscow State University,
Moscow, Russia.
The main inconvenience in using computers in chemical science courses is the necessity to spend considerable time to teach students to use various software. We are convinced that it is possible to use only one sufficiently universal program in most cases. We have chosen the Microsoft EXCEL spreadsheet. A set of problems developed to be used in the Chemistry Department of Moscow State University at seminars in Chemical Thermodynamics, Kinetics, Catalysis and Molecular Modeling are solved by students using a PC. The authors believe that this allows students to better understand the meaning of physico-chemical models which are discussed rather than simply memorizing formulas. Training students to work with EXCEL is rather easy, and provides them with the ability to solve most problems arising during the study of physical chemistry.
Spreadsheets allow students to perform numerical calculations and obtain graphs at the same working screen. Calculations can readily be performed with changes of model parameters. Speedy solution of sophisticated non-linear equations and optimization makes it possible to obtain results for direct and inverse problems similar to those encountered in research. As a result the seminars, where such problems are solved, turn into small research projects. Students have the opportunity to see the use of the textbook formulas, the effects of changes in different model parameters, the relative input of separate members of the analyzed relation, and to evaluate the methods of solving research problems. Using EXCEL it is possible, for example, to prepare tables of experimental data, to carry out calculations and to construct graphs.
It is also possible to readily perform data processing using statistical calculations on linear, logarithmic, exponential, power and polynomial functions, thus giving an estimation of the reliability of results and calculating the coefficients of the function from experimental data. Complex equations and system of equations can be solved by numerical methods. The ability to consider various physico-chemical equations, to change parameters, to represent data graphically and to carry out mathematical modeling of various processes helps to build the imagination of the students.
The developed set of problems and exercises includes support for 26 seminars and 6 tests to be taught within 2 semesters and covers the following topics: principal laws of thermodynamics; thermodynamic functions; chemical equilibrium calculation; phase rule; adsorption; statistical thermodynamics; major chemical reaction kinetics; quasi-steady state and quasi-equilibrium; auto catalysis and oscillating reactions; complex reaction's kinetics; kinetic theory of gases; the calculation of rate constants and activation energies; homogeneous, heterogeneous and enzyme catalysis. In both semester students deal with molecular modeling, calculations of molecular properties and potential energy surfaces.
To illustrate these theses we want to present four examples of EXCEL workbook.
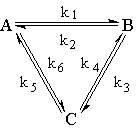
In the first workbook (ABC.XLS) the kinetic scheme for three simultaneous reversible reactions between reagents A, B and C is considered.

Varying rate constant and initial concentration of reagents in this model it is possible to illustrate a large set of basic chemical reactions (for example, AB, ABC, AB, and many others). The system of differential equations for the general case (when the initial concentations of all substances and all of the rate constants aren't equal to zero) has the analytical solution, which may be found with the help of different methods. Authors use the method of Laplace transformations, which gives sufficiently complex but analytical expressions for the concentration of substances A, B, and C as a function of time.
These analytical expressions are used in spreadsheet to calculate numerical values based on parameters of the model. Kinetic curves for reagents obtained from these values are presented on embedded chart, so students may estimate how its shape are affected by parameters' values.
This model also helps teacher to call attention of students to basic principles of chemical kinetics: quasi-steady state, quasi-equilibrium and principle of microscopic reversibility.
The second workbook (VDW.XLS) is devoted to the real gas equations, namely van der Waals equation:
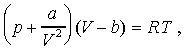
and to some aspects of its usage in physical chemistry.
p-V diagrams for this equation calculated at different temperature are presented in worksheet. Using them teacher may introduce such notions as "critical temperature" and "critical pressure". Students also have the possibility to compare shape of isotherms for ideal and van der Waals gases at different temperatures and thereby to estimate temperature limits where the ideal gas model may be used for thermodynamic and kinetic calculations.
As the last exercise students are offered to find the solution for simple numerical problem. More exactly, using van der Waals equation for a real gas at a given temperature, they must determine the value for the vapor-liquid equilibrium pressure (with the help of Maxwell's rule: p=const and S1=S2, see figure below).
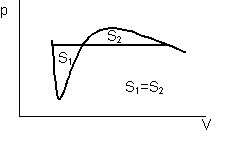
To do this they need to apply some sort of optimization technique (using the Solver Add-on) available in EXCEL.
In the third workbook (PPE.XLS) we demonstrate the use of EXCEL for constructing 3D-surface which represents the potential energy of H2 +H interaction for the linear H3 transition complex as a function of internuclear distances. London-Eyring-Polanyi approximation is used for calculation of the potential energy values (with the Morse function for interatomic interactions). All expressions needed to calculate the potential energy surface are placed on a separate worksheet as Visual Basic functions and are always accessible by students, along with the description of model parameters. Students may also modify these parameters (such as equilibrium internuclear distance, or energy of dissociation) and see what influence these changes exert on the potential energy of the molecular system under consideration.
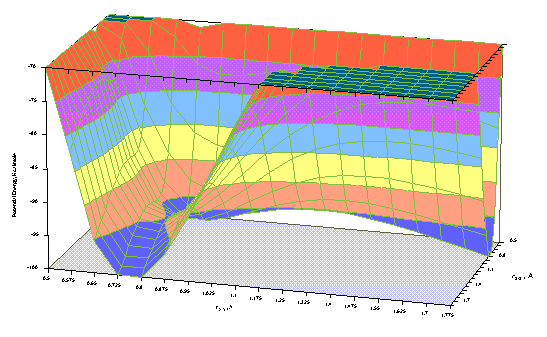
Students also have the possibility to examine 3D-chart (see figure above) for the potential energy surface from different points of view simply drugging it with the mouse.
Oscillating chemical reactions are very interesting from the educational point of view. One of the model reaction of such type, based on the scheme, originally proposed by Lotka and Volterra, is considered in the last workbook (LOTKA.XLS).
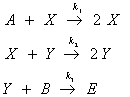
This scheme is used in biology as predator-victim model but it also has chemical analogue. Along the study of light hydrocarbons oxidation Frank-Kamenetzky have seen the periodical flashes and his kinetic model for this process is very similar to Lotka-Volterra ones.
With some simplifications it is possible to receive the analytical solution for the Lotka-Volterra problem. This workbook contains these analytical expressions and numerical values, calculated from them. The numerical values are used for graphic illustration of the reaction profile in different coordinate representations.
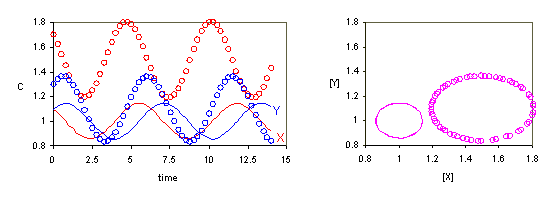
With the help of the charts presented on the
worksheet students are offered to find some "standard"
values for the parameters of considered kinetic scheme. In order
to do this they must analyse the formulas and choose the strategy
for changing values so that the curves, calculated from them,
coincide with the "standard" ones.
March, 1997